| Physical Principles: Conservation of Energy. |
| Student Prerequisites: For this demonstration, students need to be familiar with the following concepts - a.) the definitions of potential and kinetic energy in Classical mechanics, and how they tie into the Principle of Consevation of Energy, and b.) the concept of centripetal force and centripetal acceleration for a body undergoing uniform circular motion. |
| Introduction: A rather remarkable consequence of the Principle of Conservation of Energy applied to a simple pendulum dropped from a perfectly horizontal position is that the centripetal acceleration at the lowest point of the swing is independent of both, the mass of the bob, and the length of the rod/string suspending it.
In this experiment, students will combine their knowledge of the Principle of Conservation of Energy and the concept of Centripetal Acceleration to predict a simple expression for the acceleration of the pendulum at the lowest point of its swing, and hence, infer that this value is a constant, independent of the type of pendulum. The actual demonstration will subsequently prove to students, quantitatively, that the acceleration of the pendulum at the bottom of its swing is 2g, regardless of the mass and length of the pendulum used.
The primary objective of this interactive demonstration is to convince students of the fact that physical processes seldom work in isolation, so that by correctly identifying and "breaking up" a process into its sub - processes, fundamental laws may be combined to make simple, yet elegant predictions about the behavior of one or more properties of the system.
|
Description of the Demo: At the lowest point of its swing, all of the gravitational potential energy Utop that was stored in the pendulum in raising it to the top of its swing gets converted to kinetic energy Kbottom. Thus, for a pendulum with a bob of mass m on a string/rod of length r, conservation of energy yields:
Utop = Kbottom
$$mgr=\left(\frac12\right)mv^2$$
$$\Rightarrow\left(v^2\over r\right)=2g$$
Students may be reminded that an object exhibiting uniform circular motion experiences an acceleration directed toward the center of the circle, given by the expression on the left side of the equation above, so that the aim of their prediction is to find an expression for \(\left(v^2\over r\right)\), using the principle of conservation of energy.
|
| Instructions: Once students have worked on deriving expression (1) above, the instructor may proceed with the demonstration as follows. If the accelerometer is used as the mass on the pendulum, the reading at the lowest point of the swing is exactly the value of centripetal acceleration.
 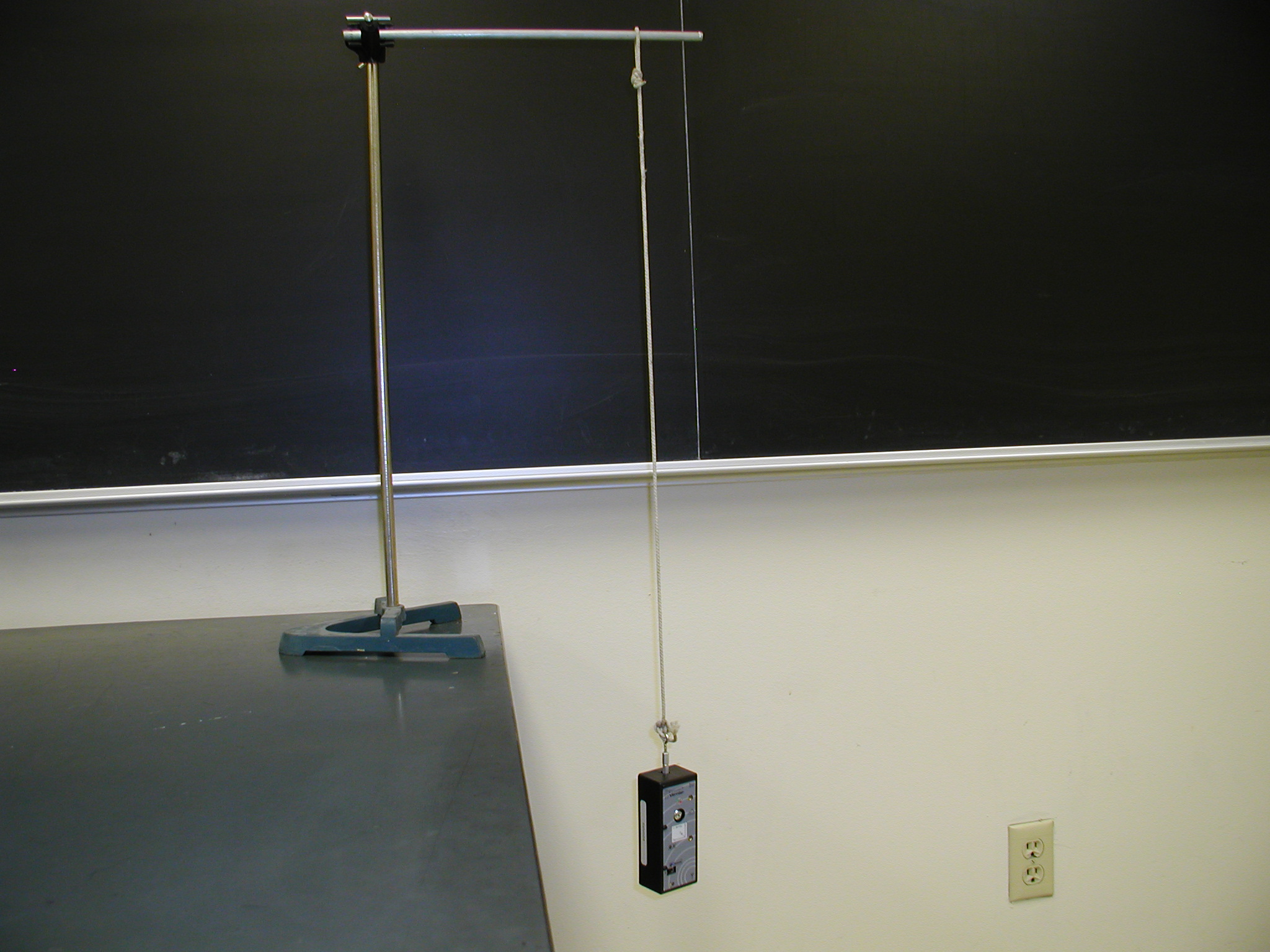
The image on the left shows the device used to measure the value of centripetal acceleration at the bottom of the swing (Vernier Wireless Dynamics Sensor System), while the image on the right shows the experimental setup, where the accelerometer is used as the bob of the pendulum.
|
| Notes to the Instructor: 1.) When the accelerometer is held perfectly horizontal, it reads approximately -g. This is because we are accelerating upward at approximately 9.8 m/s2. Thus, when calibrated properly, the accelerometer is set to read 0 when no net force acts on it, so that it reads approximately -g at the perfectly horizontal position (since it accelerates upward at an acceleration ~ g), and approximately 2g at the bottom of the swing. 2.) The first three CPS questions are intended to help students work toward the predictive parts of the demo, which are contained in the last three questions. The instructor may choose to simply remind students of the basic concepts contained in the former, and proceed to use one or more of the latter as "clicker" questions that can be supported with demonstrations. |
| Possible CPS Questions:
1. What is the energy at the perfectly horizontal position (bob released from rest)? [Question]
2. What is the energy at the bottom of the swing? [Question]
[Answer]
3. What is the speed of the bob at the bottom of the swing? [Question] [Answer]
4. What is the centripetal acceleration at the bottom of the swing? [Question] [Answer]
5. What effect does changing the mass of the bob have on the centripetal acceleration at the bottom of the swing? [Question] [Answer]
6. What effect does changing the length of the pendulum have on centripetal acceleration at the bottom of the swing? [Question] [Answer]
|
|
|
Page created by Ravi Raghunathan, Dept. of Physics & Astronomy, University of New Mexico.
|
|