| Physical Principles: Conservation of Energy, Translation and Rotation. |
| Student Prerequisites: For this demonstration, students need to be familiar with the following concepts - a.) the definitions of potential and kinetic energy in Classical mechanics, and how they tie into the Principle of Conservation of Energy, and b.) the expressions for moment of inertia of a solid cylinder, cylindrical shell, and thin spherical shell. |
| Introduction: The Principle of Conservation of Energy enables a comparison between the speeds of objects moving down an incline, provided that the moments of inertia of the objects are known. Interestingly, for a ramp of a given height (or inclination), the speeds of the objects under consideration are independent of their relative sizes and masses. In this experiment, students will combine their knowledge of the Principle of Conservation of Energy and expressions for the moments of inertia of various standard geometric shapes (such as solid cylinder, cylindrical shell and sperical shell) to derive an expression for the speed of an object as it moves down an incline. It will be seen that this speed depends on the "compactness" of the object under consideration, i.e., on the ratio of its moment of inertia to the product of its mass and the square of its radius.
The primary objective of this interactive demonstration is to show students that a seemingly complex process with a random outcome can be analyzed in a systematic way by correctly identiying and accounting for all the underlying physical processes, such that the outcome of the process can be predicted with reasonable accuracy.
|
Description of the Demo: At the bottom of the ramp, the potential energy of the ball gets converted into translational and rotational kinetic energy. Thus, for a ball of mass m with speed v at the bottom of the ramp of height h, conservation of energy yields:
Utop = Kbottom
$$mgh=\left(\frac12\right)mv^2+\left(\frac12\right)I\omega^2$$
The friction between the ramp and the objects that move down it causes a linear relation between the linear and angular speeds of the object:
$$v=r\omega$$
Thus, an expression for the linear speed of an object at the bottom of the ramp may be derived in terms of the height h of the ramp, acceleration due to gravity (g), and the moment of inertia (I), mass (m) and radius (r) of the object, by subsituting (2) into (1).
A glance at the equation so derived will show that this speed depends on the ratio of the moment of inertia of the object to the product of its mass and the square of its radius. Thus, the most "compact" object (with the smallest I to mr2 ratio) will always be the fastest down the incline.
A rather remarkable consequence of this fact is that the speeds of the objects being raced down a ramp of a given inclination are independent of their relative sizes and masses - for instance, a solid cylindrical object will always roll down a given incline faster than a hollow cylindrical object, regardless of their masses and dimensions.
|
| Instructions:The experimental setup for this demonstration is rather straightforward, as shown in the photographs below. The first photo shows the case where a solid cylindrical object is raced with a hollow cylindrical object, and the second photo shows the case where a cylindrical shell is raced with a thin spherical shell (ping pong ball).
 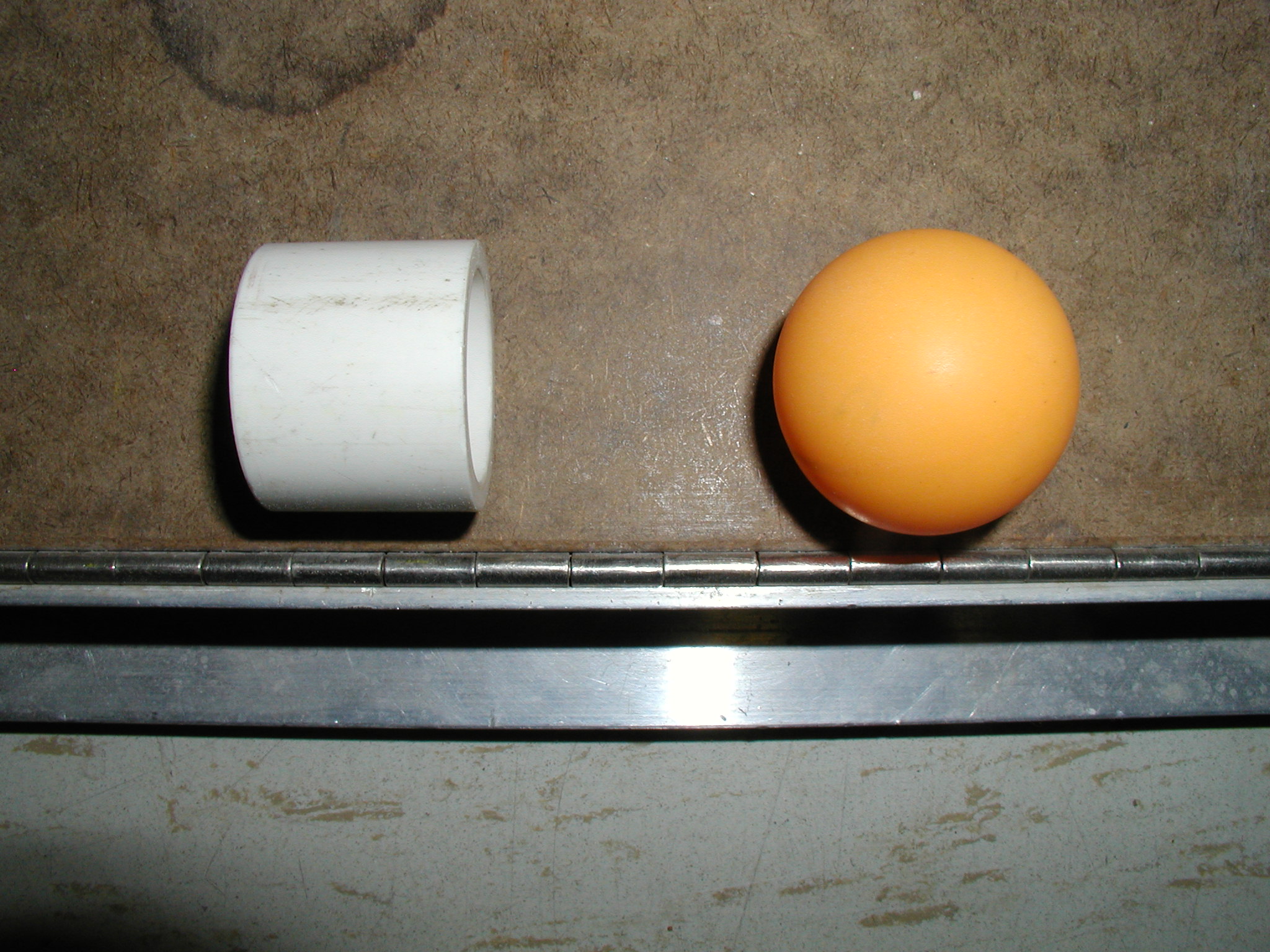
The third setup is for the case where rolling and sliding are compared - it turns out that 100 gram slotted weights work very well for this demonstration, where one of the weights is allowed to roll down the incline, while the other is allowed to slide down simply by placing it face-up or face-down on the ramp. Finally, the fourth photograph shows some of the objects that can be used in variations of this demonstration.
 
|
| Note to the Instructor: The first three CPS questions are intended to help students work toward the predictive parts of the demo, which are contained in the last three questions. The instructor may choose to simply remind students of the basic concepts contained in the former, and proceed to use one or more of the latter as "clicker" questions that can be supported with demonstrations. |
| Possible CPS Questions:
1. For an object undergoing smooth rolling motion, what is the relation between linear and angular speeds...? [Question] [Answer]
2. For an object undergoing combined translational and rotational motion, what is the equation for conservation of energy...? [Question] [Answer]
3. Combining the results in 1. and 2., what is the correct expression for linear speed...? [Question] [Answer]
4. Which do you think will win the race to the bottom of the ramp - the solid cylinder or the hollow cylinder...? [Question] [Answer]
5. Which do you think will win the race to the bottom of the ramp - the cylindrical shell or the spherical shell...? [Question] [Answer]
6. Which do you think will win the race to the bottom of the ramp - the rolling weight or the sliding weight...? [Question] [Answer]
|
|
|
Page created by Ravi Raghunathan, Dept. of Physics & Astronomy, University of New Mexico.
|
|